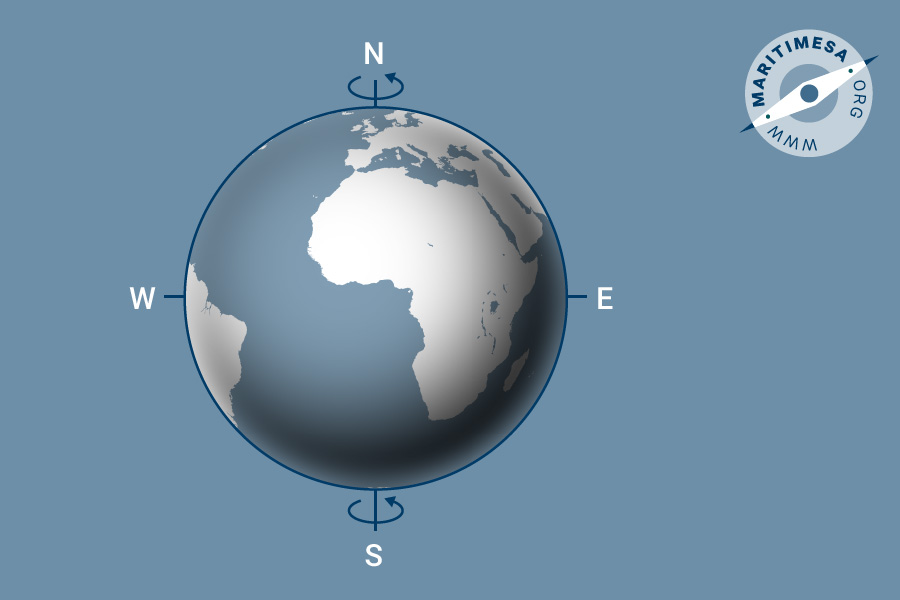
East and West. The earth is a sphere which rotates about its own axis. The direction towards which it rotates is “East” and the opposite direction is “West”. See the sketch below.
North and South. The axis of the earth has two poles. The pole to one’s left when facing east is the “North” pole and the one to the right is the “South” pole. See sketch below.
Cardinal directions
Great Circle. If you cut a sphere in half, theoretically, the circle that you would have on the flat side would be the largest that you could obtain from that sphere. This is known as a “Great Circle”. This is a very important observation for the navigator. Since the centre of the circle passes through the centre of the sphere, any section of the circle passing between two points on the surface of the sphere is the shortest distance between the two points.

Great circle
Small Circle. If the plane of a circle on the surface of a sphere does not pass through the centre of the sphere, the section is known as a “Small Circle”.

Small circle
The Equator. The great circle which lies midway between the two poles is know as the equator, ie the equator is 90° from either pole.
Meridians. These are semi-great circles joining the poles and are perpendicular to the equator.
Prime Meridian. The prime meridian is the meridian passing through the geographical position of a place called Greenwich in London, Great Britain.
Latitude and Longitude. To find the position of a point on a plane, one must find the shortest distance of that point from two lines in that plane, ie X and Y axi, which are at right angles to one another. When that point lies on a sphere one uses the same principle but the distance is measured in angular instead of linear units. The two corresponding reference lines on the earth’s surface are the equator and the Prime (Greenwich) meridian. Distances are measured in the directions governed by the earth’s rotation, ie North, South, East and West.
Latitude. The angular distance of a position on the earth’s surface measured north or south of the equator from the centre of the earth, along the meridian passing through the point, is called the “latitude” of the point. Other places having the same latitude lie on a small circle, the plane of which is parallel to that of the equator. This small circle is called a “parallel” of latitude.
Longitude. The angular distance between the meridian passing through a position on the earth’s surface and the Greenwich meridian, measured east or west of the Greenwich meridian from the centre of the earth, along the plane of the equator is called longitude.

Parallels of latitude and meridians of longitude
Difference of Latitude. The difference in latitude or “d.lat” between two places is the angular distance between the parallels of latitude passing through each place. When the two places are situated on the same side of the equator, ie in the same hemisphere (both North or both South), the smaller latitude is subtracted from the larger latitude. When the two places are situated on either side of the equator, the two latitudes are added. If the latitude of the destination is situated to the north of the departure point then the “d.lat” is named north. If it is south, it is named south.
Difference of Longitude. The difference in longitude or “d.long” between two places is the angular distance between the meridians of longitude passing through each point. If the two points both lie to the east or west of the Prime (Greenwich) meridian, then the smaller longitude is subtracted from the larger. If they are on opposite sides of the Prime meridian, then they are added. If the longitude of the destination is to the east of the departure point, then the “d.long” is named east. If it is to the west, then it is named west.
Unit of distance between two places. The most convenient unit for measuring distance between two points on the earth’s surface is based on an angular unit and is the length of a great circle arc, which subtends an angle of one minute at the centre of curvature.
Linear measurement of longitude. If you look at a three dimensional figure of the earth, you will see that the distance between any two meridians is greatest at the equator and that this diminishes uniformly until it is zero at the poles where all meridians meet. The linear measurement of a degree of longitude on the earth’s surface varies according to the latitude and cannot be taken as a standard measure of length.
Linear measurement of latitude. In contrast to that of longitude, the distance which represents a degree of latitude is approximately the same anywhere on the earth’s surface. For this reason the latitude scale is used for measuring linear distance on the earth’s surface.
Nautical mile. The nautical mile is a standard, fixed unit of distance and is equal to 1852 metres. This is used by most maritime countries as the standard measure of distance at sea.
Sea mile. The sea mile is the length of one minute of arc, measure along the meridian, in the latitude of the position. The length varies both with the latitude and with the figure of the earth in use. The sea mile is used for the scale of latitude on large scale charts, because distances are measured using the latitude graduations of the chart borders.
Geographical mile. The geographical mile is the linear measurement of one minute of arc of longitude measured on the equator. This is calculated to be 6087.2 feet or 1854.567 metres.
Knot. The unit of speed at sea is one nautical mile per hour and is referred to as one knot. There is no such thing as “knots per hour”.
Statute or land mile. This is an old imperial measure which was arbitrary introduce by Queen Elizabeth I who decreed that it was to be eight furloughs of 40 perches of 16.5 feet (5280 feet or 1760 yards). This unit is nor used in the RSA anymore.
Kilometre. The unit of distance used by many countries is a kilometre, which is equal to 1000 metres. The latter being the standard unit of measurement used by many countries that have adopted the metric system of measurement.
Cable. A cable is equal to one tenth of a nautical mile. This is not to be confused with the anchor “cable” which is a heavy chain attached to the anchor.
Direction. In order to take his ship from one point to another, the navigator must first determine the direction the destination lies from his departure point, so that by steering in that direction he will reach his destination. Direction is therefore the point on the horizon towards which a person must aim his vessel. It is essentially a line. The cardinal directions are North, South, East and West.
Bearing. In the middle of the ocean, the horizon is a circle drawn around the ship as the centre. There is nothing to distinguish one point on the circle from another. In order to proceed in a particular direction, it is necessary to refer to some datum line of fixed direction. The angle between this datum line and the direction of the point towards which the ship must proceed is called the “bearing” of that point.
True bearing. The most convenient datum is the meridian through the ship’s position, because it is the north-south line. Bearings measured from this datum are known as true bearings. They are measured clockwise from North in degrees from 0° to 360° and are always written as a three figure number, ie 000° to 360°. If the distance between the two points is relatively short, the small area of the earth’s surface traversed by the ship may be considered flat and the bearing (great circle) may be drawn as a straight line without introducing any appreciable error.

Compass
True course. The direction in which the ship moves in still water is the direction of its fore-and-aft line. The angle between the fore and aft line and the meridian running through the ship’s position is called her true course. The bearing of the ship’s head is the same as her course. The course is also given in 3 figures.

True course
Rhumb line. A rhumb line is a line on the earth’s surface that cuts all meridians at the same angle. Any one of these angles can be taken as the ship’s course. On a Mercator chart, this line appears as a straight line. On the earth’s surface and on a gnomonic chart, the line would be a curve. If the curve on the earth’s surface is continued, it would become a spiral.

Appearance of a rhumb line plotted on a Mercator chart

Appearance of a rhumb line on the earth’s surface
Departure. Departure is the distance made good in an east-west direction in sailing from one place to another along a rhumb line. Basically this is the linear distance between two meridians. An example is the distance along a parallel of latitude between two points. It is expressed in nautical miles and is equal to d.long, expressed in minutes of arc, multiplied by the cosine of the latitude. In the case where two points do not lie on the same latitude, the formula is changed slightly to read:
Departure = d.long x cosine (middle latitude).


